ایزومتری

در ریاضیات ایزومتری (انگلیسی: Isometry‎) یا طولپا به تبدیلی در فضاهای متری گفته می‌شود که فاصله نقاط را حفظ می‌کند. این تبدیل‌ها معمولاً تناظر دوسویه هستند.

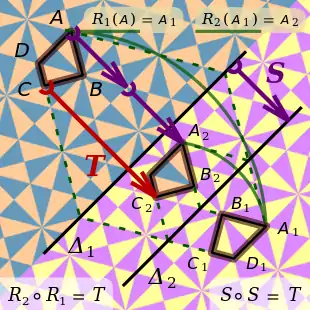
ترکیب تابع دو ایزومتری متضاد ایرومتری مستقیم است. نمایش دهندهٔ ایزومتری متضاد مانند

R 1

یا

R 2

در این تصویر. انتقال (هندسه)

T

ایزومتری مستقیم: جسم صلب.[1]

تعریف

فرض کنیم X و Y فضاهای متری با متریک‌های d_X و d_Y باشند. یک تابع ƒ : X → Y در صورتی ایزومتری تلقی می‌شود اگر برای هر a,b ∈ X رابطه زیر برقرار باشد.

d_{Y}\left(f(a),f(b)\right)=d_{X}(a,b).

[2]

مثال‌ها

هر بازتاب, انتقال و چرخش در فضای اقلیدسی یک ایزومتری است.

جستارهای وابسته

گروه فضایی
پیچ

منابع

Coxeter 1969, p. 46
Beckman, F. S.; Quarles, D. A., Jr. (1953). "On isometries of Euclidean spaces" (PDF). Proceedings of the American Mathematical Society. 4: 810–815. doi:10.2307/2032415. MR 0058193.
Let $T$ be a transformation (possibly many-valued) of $E^{n}$ ( $2\leq n<\infty$ ) into itself.
Let $d(p,q)$ be the distance between points $p$ and $q$ of $E^{n}$ , and let $Tp$ , $Tq$ be any images of $p$ and $q$ , respectively.
If there is a length $a$ > 0 such that $d(Tp,Tq)=a$ whenever $d(p,q)=a$ , then $T$ is a Euclidean transformation of $E^{n}$ onto itself.

مشارکت‌کنندگان ویکی‌پدیا. «Isometry». در دانشنامهٔ ویکی‌پدیای انگلیسی، بازبینی‌شده در ۵ مارس ۲۰۱۷.

This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.

[1] Coxeter 1969, p. 46

[2] Beckman, F. S.; Quarles, D. A., Jr. (1953). "On isometries of Euclidean spaces" (PDF). Proceedings of the American Mathematical Society. 4: 810–815. doi:10.2307/2032415. MR 0058193.
Let $T$ be a transformation (possibly many-valued) of $E^{n}$ ( $2\leq n<\infty$ ) into itself.
Let $d(p,q)$ be the distance between points $p$ and $q$ of $E^{n}$ , and let $Tp$ , $Tq$ be any images of $p$ and $q$ , respectively.
If there is a length $a$ > 0 such that $d(Tp,Tq)=a$ whenever $d(p,q)=a$ , then $T$ is a Euclidean transformation of $E^{n}$ onto itself.